Fractions Multiplication Calculator
Fraction Multiplication - work with steps
Input Data :
Fraction A = `8/3`
Fraction B = `7/2`
Objective :
Find the product of fractions?
Formula :
Solution :
`8/3\times7/2` = ?
Mutiply both numerators & denominators
`8/3\times7/2`= `(8\times7)/(3\times2)` = `56/6`
Simplify above fraction `56/6`
Common divisor of (56, 6) is 2
Divide both numerator & denominator by gcd value 2
`56/6 = frac{56\divide2}{6\divide2} = 28/3`
`8/3\times7/2 = 28/3`
Fractions multiplication calculator uses two proper or improper fractions, `a/b` and `c/d` such that `b,c,d\ne0`, and calculates the quotient for `a/b` divided by `c/d`. It is an online tool to find the quotient in the simplest form of two proper or improper fractions.
It is necessary to follow the next steps:
- Enter two fractions, `a/b` and `c/d` in the box. The numbers `a, b, c` and `d` must be integers such that `b, c` and `d` must be nonzero.
- Press the "GENERATE WORK" button to make the computation
- Fractions multiplication calculator will give the product of two numbers represented as fractions.
Output : A fraction in the simplest form
Fractions Multiplication Rule: The product of two fractions `a/b` and `c/d` is determined by the following formula
How to Multiply Fractions?
Multiplication of fractions (or any other numbers or variables) may be indicated by a multiplication sign × between two fractions, a dot between two fractions, or parentheses around one or both of fractions. For instance,
The result in a multiplication is a product. When we deal with fractions multiplication, there are three types of multiplications
Multiply fraction by fraction
To multiply two or more fractions, multiply their numerators and multiply their denominators.If the fractions have common factors in the numerators and denominators, simplify them before multiply. For instance, the product of two fractions `a/b` and `c/d` for `b,d\ne0` is
Therefore, to multiply two or more fractions it is necessary to follow three steps:
- Multiply the numerators;
- Multiply the denominators;
- Simplify the product if needed.
$$\frac{8}{3}\times\frac{7}{2}=\frac{8\times7}{3\times2}=\frac{56}{6}$$
To write the product in simplest form, find the GCF of the numerator and denominator of the product. The GCF of 56 and 6 is 2. After dividing the numerator and denominator by GCF,we get
$$\frac{56}{6}=\frac{56:2}{6:2}=\frac{27}{3}$$
Multiply fraction by whole number
Since a whole number can be rewritten as itself divided by 1, we can apply the previous rule of multiplication of a fraction by another fraction. Hence, the product of the fraction `a/b` , `b\ne0` and the whole number c, can be written in the following way
$$\frac a b\times c=\frac a b\times \frac{c}{1}$$
Multiply mixed numbers
To multiply mixed numbers, convert them to improper fractions then multiply the fractions. For example, let us multiply fractions `2\frac{2}{3}` and `\frac{7}{2}`. Since `2\frac{2}{3}` is equal to `\frac{8}{3}` then we continue multiplication steps with fractions `\frac{8}{3}` and `\frac{7}{2}` following the first case.
The similar consideration can be applied in multiplication of algebraic fractions.
The Fractions multiplication work with steps shows the complete step-by-step calculation for finding product of two fractions `8/3` and `7/2` using the multiplication rule. For any other fractions,just supply two proper or improper fractions and click on the Generate Work button. The grade school students may use this fractions multiplication calculator to generate the work, verify the results of multiplying numbers derived by hand, or do their homework problems efficiently.
Real World Problems Using Fractions Multiplication
Fractions multiplication is useful in dimensional analysis. Dimensional analysis is the process of including units of measurement in multiplication. Unit labels are considered as common factors in fraction multiplication. For example, because distance equals the rate multiplied by the time, we get
In finding an area, we usually consider the multiplication of fractions. The length and width of a rectangle represent factors, and the area of the rectangle represents their product. For example, let us find the product of `1/3` and `1/2` . Divide the rectangle into half vertically and shade the left `1/2` of the interior of the rectangle. Divide the rectangle into thirds horizontally and use a different shading to shade the bottom `1/3` of the interior. The parts that have been shaded twice is the product of these fractions, i.e `1/2\times1/3 = 1/6` . This is illustrated by the following picture:
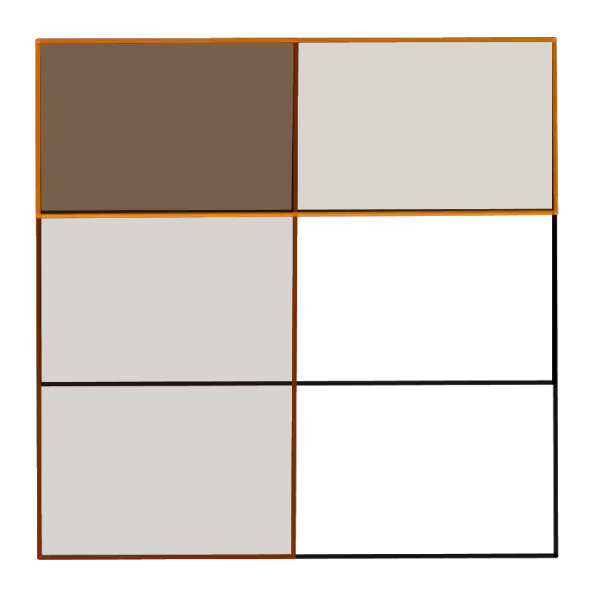
Fractions Multiplication Practice Problems
Practice Problems 1:
Find the product of `3/x` and `x^2/9` and write the result in the simplest form.
Practice Problems 2:
Ann bought 99 candies and ate `2/3` of them. How many candies did she eat?
The multiplying fractions calculator, formula, step by step calculation, real world problems and practice problems would be very useful for grade school students (K-12 education) to understand the multiplication of two or more numbers represented as fractions. Using this concept they can be able to solve complex algebraic problems and equations in the real world.
| Fractions Multiplication | |
|---|---|
| Fractions | Product |
| 1/4 times 3/4 | 3/16 |
| 8/3 times 1/3 | 8/9 |
| 2/7 times 5/3 | 10/21 |
| 4/7 times 5/6 | 10/21 |
| 4/7 times 8/3 | 32/21 |
| 1/6 times 7/3 | 7/18 |
| 1/3 times 4/7 | 4/21 |
| 9/8 times 2/5 | 9/20 |
| 9/4 times 5/6 | 15/8 |
| 1/8 times 1/4 | 1/32 |
| 8/5 times 8/7 | 64/35 |
| 3/5 times 8/3 | 8/5 |
| 7/6 times 8/9 | 28/27 |
| 2/3 times 4/3 | 8/9 |
| 8/5 times 5/7 | 8/7 |
| 7/4 times 5/2 | 35/8 |
| 9/8 times 2/7 | 9/28 |
| 8/3 times 7/3 | 56/9 |
| 9/5 times 7/8 | 63/40 |
| 7/3 times 9/4 | 21/4 |
| 4/9 times 2/7 | 8/63 |
| 1/2 times 2/3 | 1/3 |
| 5/4 times 2/7 | 5/14 |
| 9/5 times 1/9 | 1/5 |
| 7/3 times 8/7 | 8/3 |
| 7/2 times 7/5 | 49/10 |
| 5/2 times 3/2 | 15/4 |
| 1/5 times 3/4 | 3/20 |
| 7/6 times 7/2 | 49/12 |
| 7/6 times 7/8 | 49/48 |
| 1/7 times 7/4 | 1/4 |
| 1/2 times 6/5 | 3/5 |
| 8/7 times 9/7 | 72/49 |
| 6/7 times 5/6 | 5/7 |
| 2/9 times 1/3 | 2/27 |
| 3/2 times 9/8 | 27/16 |
| 4/7 times 5/3 | 20/21 |
| 8/3 times 4/5 | 32/15 |
| 7/2 times 6/5 | 21/5 |
| 9/4 times 8/9 | 2/1 |
| 8/9 times 6/5 | 16/15 |
| 7/8 times 5/9 | 35/72 |
| 9/8 times 8/9 | 1/1 |
| 1/3 times 4/9 | 4/27 |
| 8/3 times 8/5 | 64/15 |
| 1/5 times 7/4 | 7/20 |
| 1/3 times 7/3 | 7/9 |
| 8/9 times 4/7 | 32/63 |
| 3/5 times 1/2 | 3/10 |
| 1/4 times 1/5 | 1/20 |
| 1/8 times 7/4 | 7/32 |
| 7/3 times 1/2 | 7/6 |
| 1/5 times 1/9 | 1/45 |
| 1/3 times 9/5 | 3/5 |
| 2/3 times 9/5 | 6/5 |
| 7/8 times 5/7 | 5/8 |
| 4/9 times 6/7 | 8/21 |
| 5/8 times 9/4 | 45/32 |
| 3/5 times 2/9 | 2/15 |
| 1/6 times 7/6 | 7/36 |
| 9/5 times 5/6 | 3/2 |
| 6/7 times 3/7 | 18/49 |
| 5/3 times 5/4 | 25/12 |
| 8/9 times 2/3 | 16/27 |
| 8/5 times 4/3 | 32/15 |
| 7/5 times 5/8 | 7/8 |
| 9/4 times 1/4 | 9/16 |
| 6/5 times 5/4 | 3/2 |
| 7/3 times 6/5 | 14/5 |
| 5/3 times 9/4 | 15/4 |
| 1/2 times 4/5 | 2/5 |
| 9/7 times 1/2 | 9/14 |
| 1/3 times 5/9 | 5/27 |
| 8/5 times 5/4 | 2/1 |
| 4/7 times 9/5 | 36/35 |
| 2/5 times 3/4 | 3/10 |
| 7/2 times 9/4 | 63/8 |
| 5/6 times 7/2 | 35/12 |
| 5/7 times 9/4 | 45/28 |
| 5/2 times 8/9 | 20/9 |
| 2/5 times 1/2 | 1/5 |
| 3/2 times 3/8 | 9/16 |
| 5/8 times 1/4 | 5/32 |
| 1/8 times 1/7 | 1/56 |
| 4/5 times 1/3 | 4/15 |
| 5/7 times 2/7 | 10/49 |
| 8/5 times 6/7 | 48/35 |
| 3/2 times 7/6 | 7/4 |
| 5/4 times 2/9 | 5/18 |
| 3/7 times 7/3 | 1/1 |
| 5/8 times 6/7 | 15/28 |
| 2/5 times 8/7 | 16/35 |
| 1/2 times 9/5 | 9/10 |
| 5/3 times 5/2 | 25/6 |
| 6/7 times 3/5 | 18/35 |
| 4/3 times 3/5 | 4/5 |
| 7/5 times 6/7 | 6/5 |
| 9/5 times 7/3 | 21/5 |
| 7/5 times 5/7 | 1/1 |
| 5/9 times 8/3 | 40/27 |

